GMU:Non-machines/Juyoun Oh: Difference between revisions

Latest revision as of 10:27, 26 July 2024

Paidia, Hopscotch

Bauhaus-University Weimar
2023 Winter semester
Juyoun Oh

This work is inspired by the book

“The Wretched of the Screen”by Hito Styerl

“Similarities between military system and entertainment”

such as GPS

“…over the past few years, visual culture has become saturated

with aerial military and entrainment images. ”

Diagram to develop the idea

.

In recent decades, our lives have been filled with entertainment spectacles that are unwittingly based on military technology. The book I read prior to this project, "The Wretched of the Screen" by Hito Steyerl, was quite fascinating, and the sections that referenced and explored the parallels between military systems and entertainment provoked profound reflection on the ongoing conflict currently. In particular, there was a passage in the book that referred to "the ongoing Israeli-Palestinian war... (hereinafter omitted)," which was published over a decade ago, made me question whether we can actually escape the repetition of war. I would also like to say that as a citizen born and raised in South Korea, where with constant threat of conflict has exhausted me, but living in the only ceasefire country in the world has subconsciously made me more sensitive to these references than others.

This chain of thoughts made me reflect on the repetition of war and how things are progressing regardless of our efforts, and I felt helpless. It occurred to me that we are like pawns being played for a predetermined game, moving without realising that we are the "pawns" being moved, and I began to play with this helplessness in my work. Based on this reflection, I started to work on interacting with the audience. For this, I decided to make a performance which audiences will be playing or performing inspired by the traditional Korean game of “Hopscotch(Ddangddameokki in Korean)”, which is played with a ball. The rules of the game are as follows.

Draw a large square or circle.
Draw a small hand-sized circle in each corner.
Each player bounces the ball three times from their piece of land, and if it bounces back to their piece of land, that piece of land becomes theirs.
If the ball doesn't return to your land within three bounces, it's your opponent's turn.
The game continues until there is no empty land, and the player with the most land wins.

In this game, participants play with a three-dimensional object named "Trajectoid" as the "piece" of the game. However, this object is a ball that rolls along a predetermined path, symbolising the lack of alternatives to our actions. Despite the illusion of choice, the trajectory of the game is fixed, which reflects our inability to change the predetermined outcome, and the audience's efforts to deviate from the path we've already set for ourselves feel like a futile effort. The participants try their best, but despite the fact that the game is ultimately limited to a predetermined outcome, they do not realise this and continue to try to beat the game, which coincides with the feeling that we are trapped in a cycle of repeated collisions, thus the game maximises the illusion that we have the right to choose and encourages us to think about how our actions affect the determined outcome.

The current stage of the work is to experiment with an object called "Trajectoid" and a map for this game, and we presented this experimental stage at the last Winterwerkschau. The plan is to reach the first stage of completion of this work in the upcoming new semester.

REFERENCE :

Book: Hito Steyerl. The Wretched of the Screen. Sternberg Press, 2012.

Website: JooHyeon Heo. “Trajectoids: Creating a shape that rolls along a desired path” Techplore, https://techxplore.com/news/2023-09-trajectoids-desired-path.html.

What is trajectoid?

.

The concept of rolling objects traditionally brings to mind cylinders or spheres that roll in straight lines. However, researchers are exploring unique shapes like oloids and sphericons, which roll in non-linear paths. These shapes are valuable for applications such as moving objects more efficiently or mixing ingredients thoroughly. Scientists have taken this idea further by designing 3D objects, called "trajectoids," that can follow a predetermined path on a slightly inclined table without slipping. The path must be periodic, consisting of identical repeating segments, and never go uphill. The challenge was to create a shape that rolls down the table, precisely following the drawn path. To achieve this, scientists envisioned starting with a smooth basketball covered in flexible material, trimming it to form a custom shape that follows the desired path. They successfully 3D printed these trajectoids and tested them, demonstrating that trajectoids can be designed to complete two path periods for each full revolution. This two-periods-per-revolution property is significant in 3D rotations and has applications in quantum computing and quantum optics. For instance, the Bloch sphere in quantum physics represents quantum states, similar to how a rolling sphere follows a path. The trajectoid algorithm can help verify the accuracy of quantum computers by comparing changes in qubit states to the motion of a rolling trajectoid.

Additionally, this mathematical insight has implications for MRI technology. Proton spin states in MRI are analogous to trajectoid orientation. By understanding how proton spins return to their original state after specific radio wave pulses, scientists can improve MRI accuracy and disease diagnosis.

Researchers have even provided an online tool for generating 3D-printing files for custom trajectoids, encouraging further experimentation and application of this fascinating concept.

Process

First step. To design the path of the trajectoid

.

Second step. To generate the program for making trajectoid in 3D Process

Third step. To 3D print the object

Bildschirmfoto 2024-07-26 um 11.19.57.png

Forth step. Map experiment with agar

....

Exhibition for the winterwerkschau 2023

.

References

First opium war -- connection to social media as a drug

Faraday cage -- idea for a bunker

Aldous Huxley -- Brave New World (New Longman Literature), the drug SOMA

survailance cameras -- pigeon camera used in ww2

dehumanising war

@@ Line 1: / Line 1: @@
-Diagram (10.12.2023)
-[[File:Diagram10122023.png|600px]]
+== '''<big>Paidia, Hopscotch</big>''' ==
+[[File:Falling .png|thumb|left]]
+<blockquote>
-<nowiki>**</nowiki> reference for objects
-[[File:Bildschirmfoto 2024-01-08 um 18.28.22.png|left|thumb|1012x1012px|https://www.youtube.com/watch?v=2lW9HznqsVY]]
-Normally when we think of a rolling object, we tend to imagine a cylinder (like a bicycle wheel) or a sphere (like a tennis ball) that will always follow a straight path when rolling. However, the world of mathematics and science is always open to exploring new ideas and concepts. This is why researchers have been studying shapes like oloids, sphericons, and more, which do not roll in straight lines.
-All these funky shapes are really interesting to researchers as they can show us new ways to move objects around smoothly and efficiently. For example, imagine reducing the energy required to make a toy robot move, or mixing ingredients more thoroughly with a unique-looking spoon! While these peculiar shapes have been studied before, scientists have now taken it a step further.
-Consider a game where you draw a path on a tilted table – similar to tilting a pinball table to make the ball go in a particular direction. Now, try to come up with a 3D object that, when placed at the top of the table, will roll down and exactly follow that path, instead of just going straight down. There are a few other rules of this game: the table needs to be inclined slightly (and not too much), there should be no slipping during rolling, and the initial orientation of the object can be chosen at launch. Plus, the path you draw must never go uphill and must be periodic. It must also consist of identical repeating segments - somewhat like in music rhythm patterns.
-The scientists have pondered if it’s possible to find a winning strategy for this game. They wanted to know if, for any given repetitive path, it would be possible to design a shape that can be rolled to follow this path on its own. The goal was to develop a general recipe that would work not just for simple curves, but also for complicated and intertwined paths. The strangely shaped objects created for this purpose were coined as “trajectoids.”
-At first glance, it appears impossible for a 3D object to automatically follow a predetermined rolling path while navigating all the angles and curves. However, the scientists started by simplifying the problem. They envisioned starting with a perfectly smooth basketball covered in flexible, trimmable material, akin to clay. By strategically removing portions of the basketball’s cover material that made contact with the table while ensuring the basketball itself always touched the path, it is possible to gradually sculpt the object into a custom shape. This resulting shape would then magically follow the same path when rolling independently. Applying this concept, the scientists successfully devised a new method for creating trajectoids.
-These trajectoids aren’t just theoretical; the researchers 3D printed them and conducted successful experiments. They even ventured into making trajectoids that occasionally move uphill or follow self-intersecting paths. You can even try the new algorithm yourself for any path you desire, as the researchers have released an online tool for generating 3D-printing-ready files for trajectoids:
-<nowiki>https://colab.research.google.com/drive/1XZ7Lf6pZu6nzEuqt_dUCHormeSbCCMlP</nowiki>
-For a trajectoid to be successful, it must follow the periodic path indefinitely, maintaining the same orientation each time it completes a certain number of periods. The crucial aspect is how many path periods the trajectoid completes with one “full revolution,” thereby restoring its orientation. It’s highly unlikely to create a trajectoid that completes one revolution for every path period. But on the other hand, the researchers have shown that designing a trajectoid that completes two path periods for each revolution is almost always possible.
-This two-periods-per-revolution property is a manifestation of an astonishing general property of rotations in 3D space and can be used in many fields of science where phenomena can be mathematically described as 3D rotations -- for example, in quantum computing, quantum optics, and classical optics.
-In quantum physics, there’s a concept called the “Bloch sphere,” which is used to describe quantum states. These states encompass all the possible situations that a quantum system, such as a quantum bit or qubit, can be in. Just as a sphere rolling along a path provides information about its movement at specific points and orientations, the Bloch sphere represents unique qubit states, with changes in these states mirroring the motion of a rolling sphere.
+Bauhaus-University Weimar
+Winter semester
+Juyoun Oh</blockquote>
+This work is inspired by the book
+“The Wretched of the Screen”by Hito Styerl
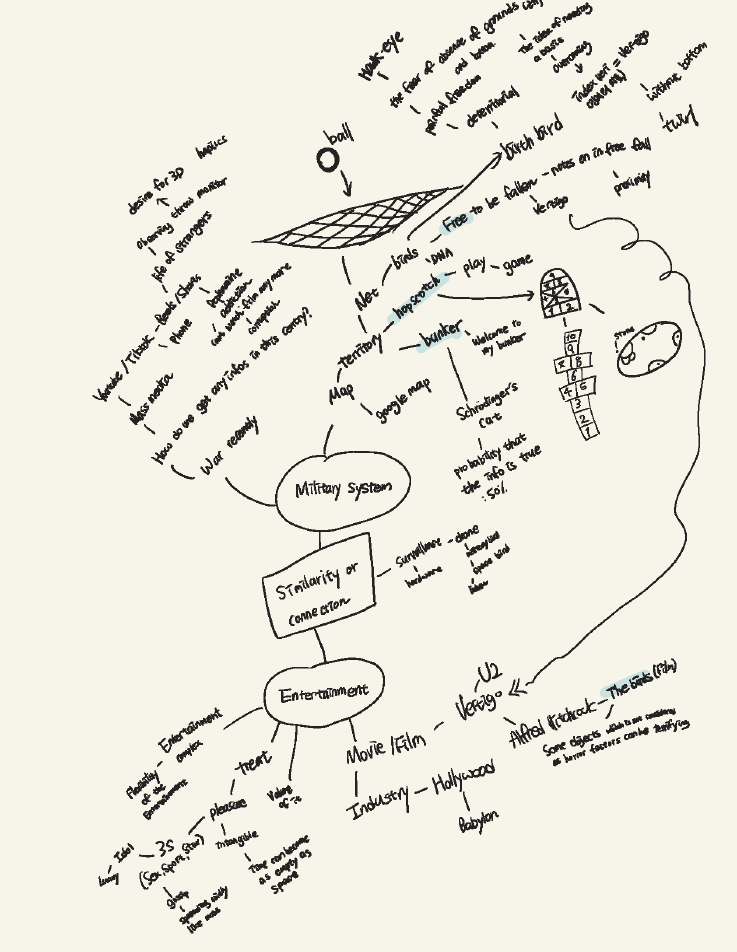
+'''“Similarities between military system and entertainment”'''
+'''such as GPS'''
+'''“…over the past few years, visual culture has become saturated'''
+'''with aerial military and entrainment images. ”'''
+[[File:Diagram10122023.png|left|thumb|952x952px]]
+''Diagram to develop the idea''
+.
+In recent decades, our lives have been filled with entertainment spectacles that are unwittingly based on military technology. The book I read prior to this project, "The Wretched of the Screen" by Hito Steyerl, was quite fascinating, and the sections that referenced and explored the parallels between military systems and entertainment provoked profound reflection on the ongoing conflict currently. In particular, there was a passage in the book that referred to "the ongoing Israeli-Palestinian war... (hereinafter omitted)," which was published over a decade ago, made me question whether we can actually escape the repetition of war. I would also like to say that as a citizen born and raised in South Korea, where with constant threat of conflict has exhausted me, but living in the only ceasefire country in the world has subconsciously made me more sensitive to these references than others.
+This chain of thoughts made me reflect on the repetition of war and how things are progressing regardless of our efforts, and I felt helpless. It occurred to me that we are like pawns being played for a predetermined game, moving without realising that we are the "pawns" being moved, and I began to play with this helplessness in my work. Based on this reflection, I started to work on interacting with the audience. For this, I decided to make a performance which audiences will be playing or performing inspired by the traditional Korean game of “Hopscotch(Ddangddameokki in Korean)”, which is played with a ball. The rules of the game are as follows.
+[[File:Image33.png|left|thumb]]
+# Draw a large square or circle.
+# Draw a small hand-sized circle in each corner.
+# Each player bounces the ball three times from their piece of land, and if it bounces back to their piece of land, that piece of land becomes theirs.
+# If the ball doesn't return to your land within three bounces, it's your opponent's turn.
+# The game continues until there is no empty land, and the player with the most land wins.
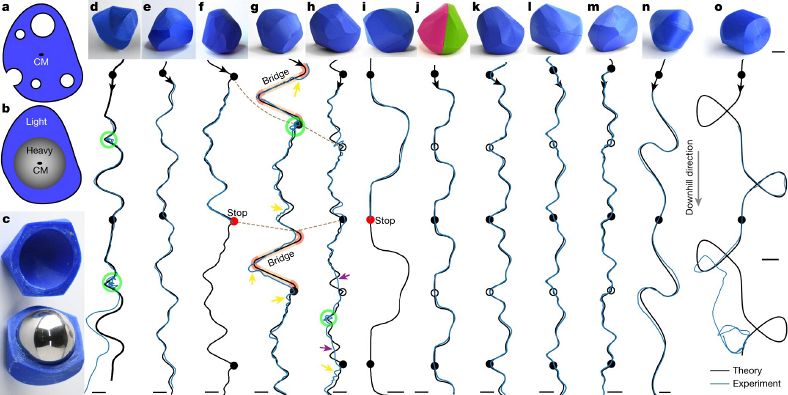
+In this game, participants play with a three-dimensional object named "Trajectoid" as the "piece" of the game. However, this object is a ball that rolls along a predetermined path, symbolising the lack of alternatives to our actions. Despite the illusion of choice, the trajectory of the game is fixed, which reflects our inability to change the predetermined outcome, and the audience's efforts to deviate from the path we've already set for ourselves feel like a futile effort. The participants try their best, but despite the fact that the game is ultimately limited to a predetermined outcome, they do not realise this and continue to try to beat the game, which coincides with the feeling that we are trapped in a cycle of repeated collisions, thus the game maximises the illusion that we have the right to choose and encourages us to think about how our actions affect the determined outcome.
+The current stage of the work is to experiment with an object called "Trajectoid" and a map for this game, and we presented this experimental stage at the last Winterwerkschau. The plan is to reach the first stage of completion of this work in the upcoming new semester.
+REFERENCE :
+'''Book:''' Hito Steyerl. The Wretched of the Screen. Sternberg Press, 2012.
+'''Website:''' JooHyeon Heo. “Trajectoids: Creating a shape that rolls along a desired path” Techplore, <nowiki>https://techxplore.com/news/2023-09-trajectoids-desired-path.html</nowiki>.
+What is '''trajectoid'''?
+[[File:Trajectoids-creating-a.jpg|left|thumb|788x788px]]
+.
+.
+The concept of rolling objects traditionally brings to mind cylinders or spheres that roll in straight lines. However, researchers are exploring unique shapes like oloids and sphericons, which roll in non-linear paths. These shapes are valuable for applications such as moving objects more efficiently or mixing ingredients thoroughly. Scientists have taken this idea further by designing 3D objects, called "trajectoids," that can follow a predetermined path on a slightly inclined table without slipping. The path must be periodic, consisting of identical repeating segments, and never go uphill. The challenge was to create a shape that rolls down the table, precisely following the drawn path. To achieve this, scientists envisioned starting with a smooth basketball covered in flexible material, trimming it to form a custom shape that follows the desired path. They successfully 3D printed these trajectoids and tested them, demonstrating that trajectoids can be designed to complete two path periods for each full revolution. This two-periods-per-revolution property is significant in 3D rotations and has applications in quantum computing and quantum optics. For instance, the Bloch sphere in quantum physics represents quantum states, similar to how a rolling sphere follows a path. The trajectoid algorithm can help verify the accuracy of quantum computers by comparing changes in qubit states to the motion of a rolling trajectoid.
+Additionally, this mathematical insight has implications for MRI technology. Proton spin states in MRI are analogous to trajectoid orientation. By understanding how proton spins return to their original state after specific radio wave pulses, scientists can improve MRI accuracy and disease diagnosis.
+Researchers have even provided an online tool for generating 3D-printing files for custom trajectoids, encouraging further experimentation and application of this fascinating concept.
+'''Process'''
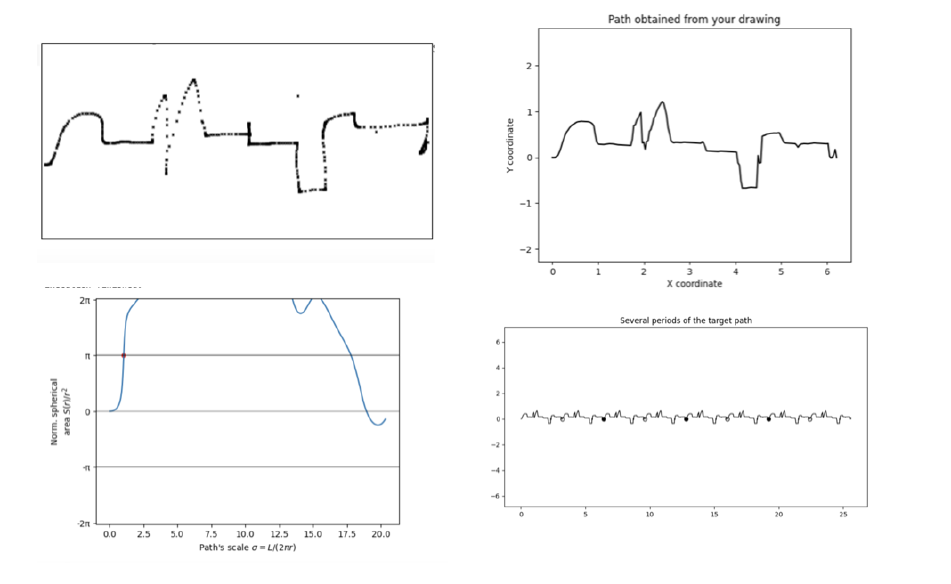
+First step. To design the '''path''' of the trajectoid
+[[File:Firststep.png|left|thumb|951x951px]]
+.
+.
+.
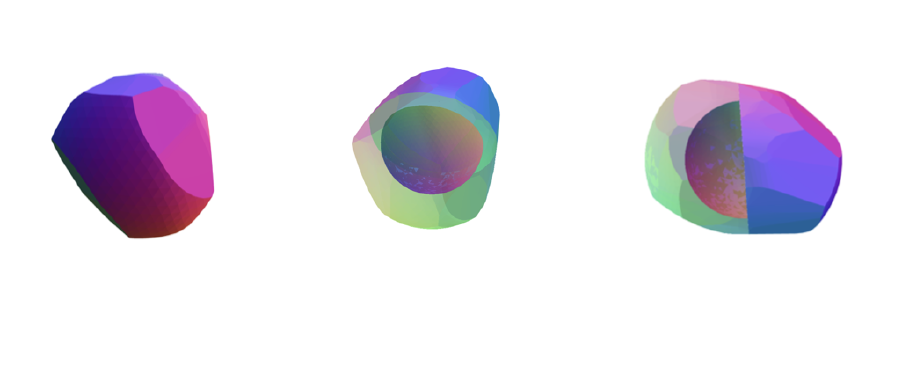
+Second step.  To generate the program for making trajectoid in '''3D   Process'''
+[[File:Bildschirmfoto 2024-07-26 um 11.19.51.png|left|thumb|902x902px]]
+Third step. To 3D print the object
+[[File:Bildschirmfoto 2024-07-26 um 11.19.57.png|left|thumb|1022x1022px]]
+Forth step. Map experiment with agar
+[[File:Bildschirmfoto 2024-07-26 um 11.20.01.png|left|thumb|975x975px|....]]
+'''Exhibition for the winterwerkschau 2023'''
+[[File:Image-exbihition1.png|left|thumb|745x745px]]
+[[File:Image-winterwerkschau.png|left|thumb|841x841px]]
+.
+.
+.
-Given this mathematical similarity, scientists can use the same algorithm for designing trajectoid to help verify the accuracy of quantum computers. Scientists often assess this accuracy by examining how closely a point on the Bloch sphere returns to its original position after specific actions, much like when a trajectoid completes one full revolution and restores its orientation after passing two path periods.
-The science of crafting customizable trajectoid is also related to another seemingly unrelated field – disease diagnosis via MRI. “Spin” is a fundamental property of particles like protons, which make up hydrogen atoms in our bodies. Protons behave as tiny magnets, with their “spin” dictating the orientation of their magnetic ‘north’. This property is crucial for Magnetic Resonance Imaging (MRI) machines used in hospitals for patient scans.
-To connect this with the Bloch sphere, consider that each point and orientation on the Bloch sphere represents a unique proton spin state, much like our trajectoid’s orientation. MRI machines use powerful magnetic fields to align these proton magnets within the body in one direction. Subsequently, they employ radio waves to disrupt this alignment. As the protons naturally realign themselves, they emit signals that can be measured and used to create detailed internal images.
-Understanding how each proton returns to its original point on the Bloch sphere helps scientists differentiate human tissues and identify abnormalities. Mathematically, the proton spin state is analogous to the trajectoid’s orientation, the radio waves represent the path, and the changes in proton spin caused by the radio waves are equivalent to the rolling motion of the trajectoid along the path.
-The mathematics behind the trajectoid algorithm reveals how any given MRI radio wave pulse can be finely tuned, such that repeating the pulse twice in succession restores all proton spins to their original state. This insight could potentially enhance MRI machines and improve disease diagnoses with greater accuracy.
@@ Line 46: / Line 269: @@
-== References ==
-First opium war -- connection to social media as a drug
+.
+.
+== References ==
+First opium war -- connection to social media as a drug